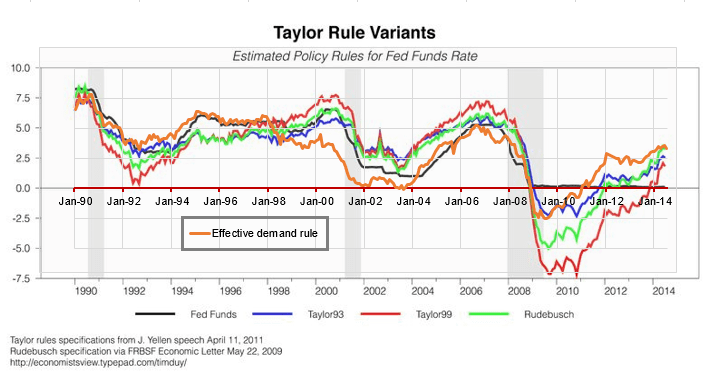
I posted a graph where I overlaid the Effective Demand rule over the Taylor rule variations done by Tim Duy.
You can see differences between the rules since the crisis. Why the difference? And does the difference matter?
Basic Taylor rule
Target Fed rate = natural real rate + inflation + 0.5*(inflation – inflation target) + 0.5*(output – output target)
When inflation or output is below target, the target Fed rate will be lower. The problem we have seen with the Taylor rule is the trouble that the CBO has had with estimating potential output. The CBO has had to continually adjust downward potential output. There are disagreements to what potential output really is.
Rudebusch Variation of Taylor rule
On the other hand, the Glenn Rudebusch rule which is a variation of the Taylor rule measures unemployment to a Natural rate of unemployment.
Rudebusch target Fed rate = natural real rate + inflation target + 1.3*(inflation – inflation target of 2%) – 1.9*(unemployment rate – natural unemployment rate target)
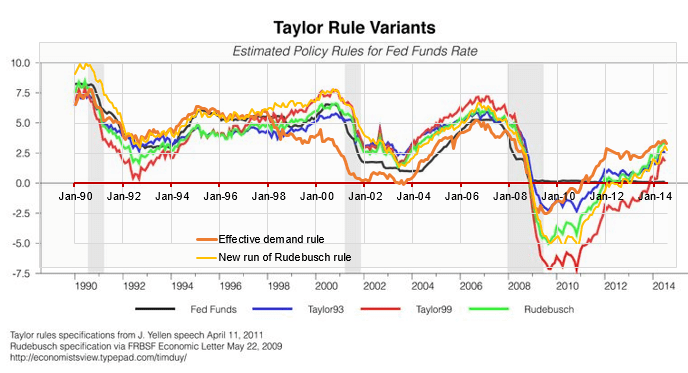
Let’s test data through this equation. (I use the Short-term NAIRU data from FRED for natural unemployment rate target. CPI less food & energy for inflation.) I now overlay my new run of the Rudebusch rule on the above graph (yellow line).
The yellow line and the green line (Tim Duy’s Rudebusch run) follow each other pretty well since 2002.
Effective Demand Rule
You will notice that the target rate of the Effective Demand rule rose faster than the Rudebusch rule after the crisis. Why? Well, it is because the Effective Demand rule measures the utilization of labor AND capital (TFUR in equation below), not just labor like the Rudebusch rule. After the crisis capacity utilization rose much faster than unemployment fell. So while the Rudebusch rule lagged behind with unemployment, the ED rule rose by following both capacity utilization and unemployment.
ED target Fed rate = z*(TFUR2 + ELS2) – (1 – z)*(TFUR + ELS) + inflation target + 1.3*(current inflation – inflation target)
z = (2*ELS + NR)/(2*(ELS2 + ELS))
TFUR = Total Factor Utilization Rate, (capacity utilization * (1 – unemployment rate)).
ELS = Effective Labor Share is Non-farm Labor Share: Business sector * 0.765. This value of labor share is the only value that makes the equation work. It is the basic factor to determine effective demand. ELS is determined by labor share’s cyclical relationship with capacity utilization. ELS represents the central tendency in the cyclical movement of capacity utilization.
NR = Natural real rate of interest.
Inflation target = 2.0% (assumed to be 3% before 1980 in the graph above.)
Current inflation = The monthly value of CPI (less food & energy) is used in the graph.
1.3 coefficient = To give the Fed rate leverage when inflation gets off target. Fed rate would change 1.3x more than inflation is off target. Same value as used in the Rudebusch rule.
Is it better to include capacity utilization in a rule for the Fed rate?
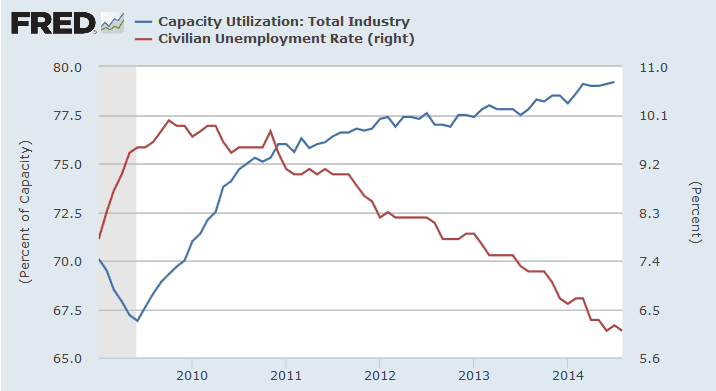
The rapid rise in capacity utilization was increasing production and using up economic slack. If you just include unemployment in a policy rate rule, you would not see that effect.
Capital and labor are both directly related in the productive capacity of the economy to produce what is demanded. Some say that capital is being used more due to new technologies and replacing labor. If so, we must have a measure of capacity utilization along with unemployment in a policy rule.
Capacity utilization has been moving steadily on its trend line since the beginning of 2011, and so has unemployment. They are both heading toward a natural limit together. We may see utilization of capacity drop while utilization of labor rises, which is a sign that they are reaching their composite natural limit to meet effective demand.
It may also be reasonable to say that keeping nominal rates low makes the cost of capital cheaper and thus that much more desirable than hiring labor. In such a case, a higher Fed nominal rate would have reduced labor’s advantage against capital.
So, I would say… definitely yes. A rule for a target Fed rate should include capacity utilization or another measure of capital utilization.